Next: kernels, cokernels, etc. on Up: , , and the Previous: sheafication of a sheaf
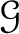 be a presheaf on a topological space
be a presheaf on a topological space 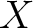 .
Let
.
Let  be a point. We define the stalk of
be a point. We define the stalk of
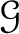 on
on  as
as

It should be noted at this stage that
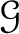 be a presheaf on a topological space
be a presheaf on a topological space 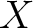 .
The natural map
.
The natural map

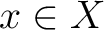 .
.