


Next: About this document ...
�����Ƚ������� No.9
����������Ȥ��ˡ��֥ۥƥ�ҥ�٥�ȡפΤ褦�ʲ���Ǥ���ΤǤ�����
���β��Ǥϡ����ͤϡ��ֶ��������ʤ����ȡפ��б�����
ñ�ͤϡ��ֳ������ļ���(ñ�ͤǤʤ����Ȥϡ�
�������������뤳��)���б�����ΤǤ�����
���ͤ�ñ�ͤ�¸�ߤϡ��Ͻ���Ƚ�����θ���¿���ȴط����Ƥ���ΤǤ�����
&dotfill#dotfill;
��9���ܤμ��� :

���� 9.1 (��)
����
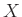
,
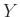
��Ϳ�����Ƥ���Ȥ��롣
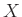
�Τ��Τ��Τθ�
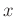
������
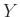
�Υ��ԡ�
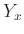
���Ѱդ���С�
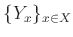
�ϤҤȤĤν����²�Ǥ��롣
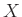
����
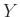
�ؤμ���
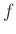
��
�θ�
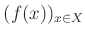
��Ʊ��뤵��롣���ʤ����
ľ�ѽ���
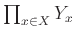
��
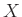
����
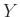
�ؤμ������Τν����Ʊ���Ǥ��롣
��� 9.2 (��)
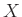
����
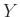
�ؤμ��������ΤΤʤ������
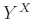
�Ƚ�����Ϥޤ�
�Ƚ��⤢�롣
���� 9.3 (�������)
���Ǥʤ�����Ф��꤫��ʤ뽸��²
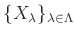
��
�������ơ�
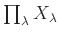
�϶��ǤϤʤ���
����������ȡ�̵�¸Ĥζ��Ǥʤ����礿��
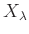
���顢���ä����˰�ĤŤ�
������Ф����Ȥ���ǽ�Ǥ��롣
���������
��� 9.4
����
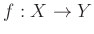
��
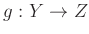
��Ϳ�����Ƥ���Ȥ��롣
���ΤȤ���
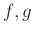
��
��������
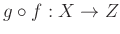
��
��������롣
����̿��ϴ�ñ�ǤϤ��뤬ͭ�ѤǤ��롣���Ѿ�Ϥ��Τ褦��̿�꤬���뤳�Ȥ���
�������Ƥ����ơ���������Ƭ����dzΤ����Τ�������������
��� 9.6
����
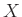
���Ф��ơ�����

��
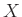
��
���������Ȥ�����
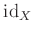
��ɽ����
���̿��⡢
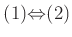 �ʳ��Ϥ������ٳ�ǧ������ɤ���
�ʳ��Ϥ������ٳ�ǧ������ɤ���
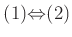 ���ä˽��פǤ��롣
���ä˽��פǤ��롣
��� 9.8
�¿�
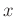
���Ф��ơ�
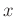
��Ķ���ʤ��褦�������Τ�������Τ�Τ�
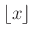
�Ƚ�(floor of
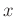
���ɤࡣ)��
�㤨�С�
�Ǥ��롣�ޤ���Ǥ�դ�����
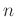
���Ф��ơ�
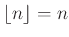
�Ǥ��롣
���̤ˡ��¿� 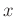 ������
������ 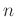 ���Ф��ơ�
���Ф��ơ�
�ˤ����դ��Ƥ��������Τ�
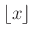 �Τ��Ȥ�
�Τ��Ȥ� ![$ [x]$](img42.png) ��
�ơ��֥���������פȸƤ֤��Ȥ�¿���ä�����
���� floor �Τۤ����̤꤬�ɤ��ʤ�ĤĤ���褦�Ǥ��롣
��
�ơ��֥���������פȸƤ֤��Ȥ�¿���ä�����
���� floor �Τۤ����̤꤬�ɤ��ʤ�ĤĤ���褦�Ǥ��롣
���� 9.2
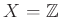
,
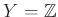
�Ȥ�����
����
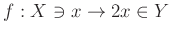
��
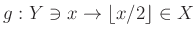
�ˤ������ơ�
-
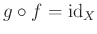 �Ǥ��뤳�Ȥ��ʤ�����
�Ǥ��뤳�Ȥ��ʤ�����
-
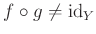 �Ǥ��뤳�Ȥ��ʤ�����
�Ǥ��뤳�Ȥ��ʤ�����
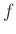 ,
, 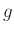 �Ϥ��줾�����͡�ñ�͡���ñ�ͤ���������
�Ϥ��줾�����͡�ñ�͡���ñ�ͤ���������
���� 9.3
![$ X={\mathbb{C}}[t] $](img48.png)
(ʣ�ǿ�������
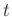
���ѿ��Ȥ���¿�༰�����ΤΤʤ�����),
![$ Y={\mathbb{C}}[t]$](img50.png)
�Ȥ�����
����
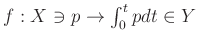
��
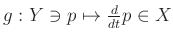
�ˤ������ơ�
-
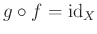 �Ǥ��뤳�Ȥ��ʤ�����
�Ǥ��뤳�Ȥ��ʤ�����
-
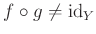 �Ǥ��뤳�Ȥ��ʤ�����
�Ǥ��뤳�Ȥ��ʤ�����
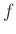 ,
, 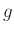 �Ϥ��줾�����͡�ñ�͡���ñ�ͤ���������
�Ϥ��줾�����͡�ñ�͡���ñ�ͤ���������



Next: About this document ...
2012-07-12
![]()
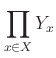
![]() ������
������ ![]() ���Ф��ơ�
���Ф��ơ�