For any subset
Then we may topologize
We refer to the topology as the Zariski topology.
Yoshifumi Tsuchimoto
For any subset
Then we may topologize
We refer to the topology as the Zariski topology.
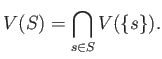
It is continuous with respect to the Zariski topology.
is a closed map with respect to the Zariski topology.
holds.
of irreducible subsets of
We define the Krull dimension of a ring ![]() as the dimension of
as the dimension of
![]() .
.