definition of connections on quasi coherent sheaves

Let $X$ be a separated scheme over $S$ . Let $\mathcal{V}$ be a quasi coherent sheaf on $X$ . Let $p_1^{(1)},p_2^{(1)}:\Delta^{(1)}_{X/S} \to X$ be the canonical projections.

There are a several equivalent ways to give a ``connection on $\mathcal{V}$ ".

One way is to provide an isomorphism

$$P: (p_1^{(1)})^*\mathcal{V}\cong (p_2^{(1)}) ^* \mathcal{V}$$

such that $P\vert _{\Delta_{X/S}}=\operatorname{id}$ . (Since the structure sheaf of $\Delta^{(1)}_{X/S}$ is an extension of that of $\Delta_{X/S}$ by nilpotents, we may easily prove that the term ``isomorphism'' here may be safely replaced by a term ``homomorphism''.)

By the adjoint relation, we see that giving $P$ is equivalent to giving an $\mathcal{O}_X$ -linear homomorphism

$$D: \mathcal{V}\to (p_1^{(1)})_* (p_2^{(1)}) ^* \mathcal{V} =\mathcal J_1(\mathcal{V})$$

such that the composition

$$\mathcal{V}\overset{D}{\to} \mathcal J_1(\mathcal{V}) \to \mathcal{V}$$

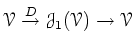
is equal to identity.

Now let us call $\nabla=jet_1-D$ ``the covariant derivation''. Then $\nabla$ is $\mathcal{O}_S$ -linear homomorphism

$$\nabla: \mathcal{V}\to \Omega^1_{X/S} \otimes \mathcal{V}.$$

In terms of the covariant derivation $\nabla$ , the $\mathcal{O}_X$ -linear is expressed as the following identity.

$$\nabla (f m)=f \nabla(m)+d f\otimes m \quad (f \in \mathcal{O}_X, m\in \mathcal{V})$$ (Co)

Let us put it in terms of rings and modules. Let $X=\operatorname{Spec}(B)$ and $S=\operatorname{Spec}(A)$ . $I=I_{\Delta}$ be the defining ideal of the diagonal $X\times_S X$ .

The $\mathcal{O}_X$ -linear homomorphism $D$ corresponds to a $B$ -module homomorphism

$$D:M\to ((B\otimes_A B)/I^2) \otimes_B M.$$

such that

$$1\otimes m -D(m) \in (I/I^2)\otimes_B M (\cong \Omega^1_{B/A} \otimes_B M)$$

holds for all $m\in M$ . The left hand side of the above formula is $\nabla(m)$ . Namely,

$$\nabla(m)=jet_1(m)-D(m)=1\otimes m -D(m).$$

Let us verify the identity (Co).

$$\nabla(fm)=1\otimes fm -D(fm)$$

$$=1\otimes fm -f D(m)$$

$$=(1\otimes f -f\otimes 1) m+f(1\otimes m -D m)$$

$$=d f \otimes m + f \nabla (m)$$