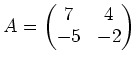: ����ʸ��ˤĤ���...
����� A No.7����
 �ԴĤν�Ʊ������������ˡ��
�ԴĤν�Ʊ������������ˡ��
�� 7.1 (��Ʊ�������δ�����1)
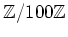
����
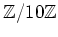
�ؤμ���
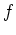
��
![% latex2html id marker 1002
$\displaystyle f([n]_{100})=[n]_{10} \quad \quad ($](img5.png)
$[?]_n$ �� $$
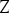
$/n$
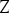
$$ �ˤ�����
$?$ ��Ʊ����
������ȡ����Τ��Ȥ�ʬ���롣
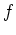 �ϼ����Ȥ��Ƥ��ޤ��������Ƥ��롣
���ʤ����
�ϼ����Ȥ��Ƥ��ޤ��������Ƥ��롣
���ʤ����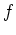 ���������ɽ���ΤȤ����ˤ��ʤ���
���������ɽ���ΤȤ����ˤ��ʤ���
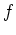 �ϴĤν�Ʊ���Ǥ��롣
�ϴĤν�Ʊ���Ǥ��롣
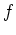 ������
������
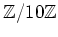 ���ΤǤ��롣
���ΤǤ��롣
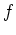 �γˤ�
�γˤ�
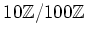 �Ǥ��롣
�Ǥ��롣
��äơ���Ʊ�������ˤ�ꡢ
����������롣
�� 7.2 (��Ʊ�������α����㣱)
![$ {\mbox{${\mathbb{Z}}$}}[X]$](img10.png)
����
![% latex2html id marker 1026
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{14}]$](img11.png)
�ؤμ���
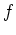
��
������ȡ����Τ��Ȥ�ʬ���롣
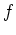 �ϼ����Ȥ��Ƥ��ޤ��������Ƥ��롣
���ʤ����
�ϼ����Ȥ��Ƥ��ޤ��������Ƥ��롣
���ʤ����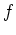 ������
������
![% latex2html id marker 1036
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{14}]$](img13.png) ����Ϥ߽Ф��ʤ���
����Ϥ߽Ф��ʤ���
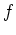 �ϴĤν�Ʊ���Ǥ��롣
�ϴĤν�Ʊ���Ǥ��롣
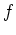 ������
������
![% latex2html id marker 1042
$ {\mbox{${\mathbb{Z}}$}}[\sqrt{14}]$](img14.png) ���ΤǤ��롣
���ΤǤ��롣
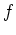 �γˤ�
�γˤ�
![% latex2html id marker 1046
$ (X^2-14){\mbox{${\mathbb{Z}}$}}[\sqrt{14}]$](img15.png) �Ǥ��롣
�Ǥ��롣
��äơ���Ʊ�������ˤ�ꡢ
����������롣
�� 7.3 (��Ʊ�������α����㣲)
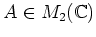
��
����ᡢ
![$ {\mathbb{C}}[X]$](img19.png)
����
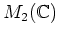
�ؤμ���
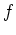
��
������ȡ����Τ��Ȥ�ʬ���롣
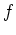 �ϴĤν�Ʊ���Ǥ��롣
�ϴĤν�Ʊ���Ǥ��롣
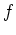 ������
�Ǥ��롣
������
�Ǥ��롣
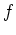 �γˤ�
�γˤ�
![$ (X^2-8 X-8){\mathbb{C}}[X]$](img23.png) �Ǥ��롣
�Ǥ��롣
��äơ���Ʊ�������ˤ�ꡢ
����������롣
����ݡ�������
�Ĥ��Τ�����������Ʋʤ�����
(���¡����ιֵ��ν�λ���ޤǡ�)
- (I).
- �ĤȤ��Ƥ�Ʊ��
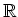
![$ [X]/(X^2+1)$](img26.png)
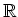
![$ [X] \cong {\mathbb{C}}$](img27.png) ��
¸�ߤ��뤳�Ȥ��ʤ�����
��
¸�ߤ��뤳�Ȥ��ʤ�����
- (II).
-
���֤��Ȥ���
-
![$ {\mathbb{C}}[A] \cong {\mathbb{C}}[X]/(X^2-5X+6){\mathbb{C}}[X]$](img29.png) �Ǥ��뤳�Ȥ��ʤ�����
�Ǥ��뤳�Ȥ��ʤ�����
-
![$ {\mathbb{C}}[X]/(X^2-5X+6){\mathbb{C}}[X]$](img30.png) �� 0 �Ǥʤ������Ҥ��Ĥ����ʤ�����
�� 0 �Ǥʤ������Ҥ��Ĥ����ʤ�����
-
![$ {\mathbb{C}}[A]$](img31.png) �� 0 �Ǥʤ������Ҥ��Ĥ����ʤ�����
�� 0 �Ǥʤ������Ҥ��Ĥ����ʤ�����
ʿ��17ǯ11��24��
![]() �ԴĤν�Ʊ������������ˡ��
�ԴĤν�Ʊ������������ˡ��
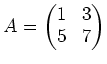
![$\displaystyle {\mathbb{C}}[A]=
{\mathbb{C}}A+{\mathbb{C}}E= \{k A+ lE ; k,l \in...
...in{pmatrix}
k+l &3k \\
5k &7k+l
\end{pmatrix}; k,l \in {\mathbb{C}}
\right \}
$](img22.png)