


: ����ʸ��ˤĤ���...
���������II ���� No.14
�����Υơ���:

�ؿ��Ĥ�������ɲä�������
��� 14.1
![$ {\mathbb{C}}[x]$](img2.png)
��
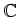
��
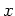
���ɲä��ƤǤ���ġ����ʤ��
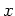
�ˤĤ��Ƥ�
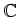
������¿�༰���ΤΤʤ��ġ�
![$ {\mathbb{C}}[[x]]$](img5.png)
��
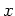
�η���Ū�٤�����Τʤ��ĤǤ��ä���
![$ {\mathbb{C}}[[x]]$](img5.png)
����ʬ��
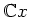
��
![$\displaystyle {\mathbb{C}}\convergent{x}=\{f=\sum_{i=0}^\infty a_i x^i\in {\mathbb{C}}[[x]];$](img7.png)
$f$ �μ�«Ⱦ�¤���
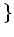
���������
��μ�«�٤�����ĤȸƤ֡���
����ǥå����ε���ϡ��ؿ���
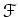 ���������Ƽ��Τ褦�˽Ƥ���
�Τ��褤��
���������Ƽ��Τ褦�˽Ƥ���
�Τ��褤��
��� 14.2 (���13.2�Ʒ�)
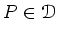
�Ȥ��롣
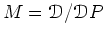
�Ȥ����ơ�
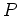
��
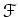
��Υ���ǥå�����
��������롣(â����Ĥμ������Ȥ��ͭ�¼����ΤȤ���)
�ֵܹ��λ��ͽ�ֲ÷����áפˤϥޥ륰��������(161�ڡ���������10.1)��
�Ƥ��롣
���� 14.1 (�ޥ륰���)
![$ P\in \mathcal D={\mathbb{C}}[x,\partial_x]$](img14.png)
��
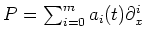
�Ƚ��Ȥ���
-
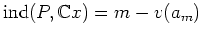
-
![% latex2html id marker 838
$ \operatorname{ind}(P,{\mathbb{C}}[[x]])=\underset {0\leq i \leq m}{\max}(i-v(a_i))$](img17.png)
(���
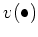
��
�������ΰ̿���魯��)
���ιֵ��ǤϤ��������ξ����ϽҤ٤ʤ�����
������ˤĤ��ƥ���ǥå������ɤ��ʤäƤ��뤫��
�Ҥ٤�����
�� 14.1
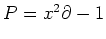
�ˤ������ơ�
-
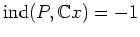
-
![$ \operatorname{ind}(P,{\mathbb{C}}[[x]])=0$](img22.png)
�ºݡ�������ˤĤ��Ƥ�
��
�Ϥ����ˤ狼�롣
���ݥ���Ȥǡ��������ʬ�������η���Ū�ʲ�ɬ�������«���ʤ����Ȥ�
�б����Ƥ��롣
ʿ��16ǯ2��19��
![]()
![$\displaystyle {\mathbb{C}}\convergent{x}=\{f=\sum_{i=0}^\infty a_i x^i\in {\mathbb{C}}[[x]];$](img7.png) $f$ �μ�«Ⱦ�¤���
$f$ �μ�«Ⱦ�¤��� ![]() ���������Ƽ��Τ褦�˽Ƥ���
�Τ��褤��
���������Ƽ��Τ褦�˽Ƥ���
�Τ��褤��